The T-Module in SynergeticsThe Rhombic Triacontahedron of 5-tetravolumes |
|
|
In Synergetics, the rhombic triacontahedron consisting of 120 T-modules weighs in at volume 5, meaning each of the 120 Ts has a volume of 1/24, equivalent to the volumes of the A and B modules. The rhombic triacontahedron with this volume has a body center to face center radius of length h, which is also the altitude of the T-module. It turns out that h is just shy of being the Prime Vector Radius (the radius of an IVM sphere). |
 |
|
The calculations below show one way to calculate the volume of the rhombic triacontahedron, using the usual 1/3 x base x height method, multiplying by 120, and then multiplying the result by Syn3, the third power of the Synergetics Constant. This last step is required to adjust for the fact that Synergetics uses a different model of third powering, i.e. sets the tetrahedron defined by 4 unit-radius spheres packed together as volumetric unity. |
|
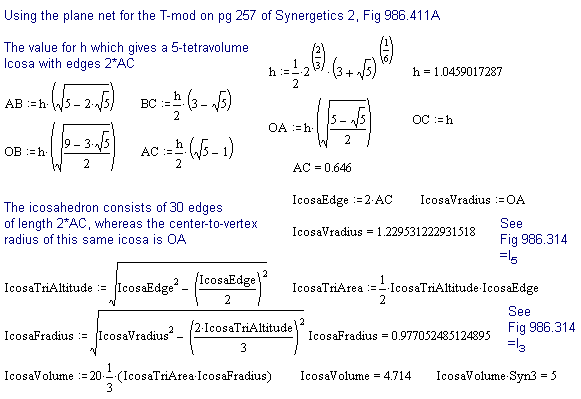
 The Icosahedron of 5-tetravolumes |
|
|
The calculations below (which use the same T-mod plane net as above) are performed as a check on the derived value for h that will produce the desired result. The values obtained for the resulting icosa's body center to vertex and body center to mid face dimensions provide a check for Fig. 986.314 in Synergetics 2 (and a few more digits of accuracy), which was originally developed by Fuller's associates Robert Grip and Chris Kitrick. Again, the Syn3 is used to convert the resulting volume into synergetics' tetravolumes. |
 |
 |
|
Ongoing ExplorationsSince Fuller's time, further work on the T-module has been undertaken by David Koski.Koski has shown that:
Note: Gerald's applet values the distance between adjacent IVM sphere centers as one, so, for example, you would need to divide the above plane net values in half in order to get volume = 5 in each instance. Synergetics on the
Web |
|